Site Map: MAIN / A Reader's Journal, Vol. 2 This Page
![Click to return to ARJ Page, File Photo of Denis Guedj [ or from [Credits for source of photo]]](http://www.doyletics.com/arj/parroaut.jpg)
A READER'S JOURNAL
The Parrots Theorem
A Novel
by
Denis Guedj
Translated by Frank Wynne
Published by Weidenfeld&Nicolson/UK in 2000
A Book Review by Bobby Matherne ©2007
Like Us? Subscribe to Receive a Monthly Email
Reminder of New Reviews & New DIGESTWORLD Issues CLICK
How can a novel be a runaway bestseller in France and not be heard of in the United States? It must have something to do with mathematics, one might guess, and one would be right. Within the cover of this 344 page novel, the author weaves into the fabric of an adventure story the fascinating history of mathematics, covering every major mathematician's life and contribution. This is not likely to pull viewers away from Survivor, Dancing with Celebrities, Bridezilla, or any popular sitcom on television these days. The author is a professor of the History of Science in Paris and must be delighted at the response of his country to his tale of the origin of mathematics up to the present day.
This is the second novel in a row I've read where the curious piece of mathematical lore known as Pi figures prominently(1). Our hero and mathematics learner and teacher is Pierre Ruche, and his name gives no clue as to Pi (π) when we say his name, but visually we note it is contained in the first two letters, Pi. But if you take the equation for one half of the circumference of a circle, πR, and pronounce it in French, you get the auditory equivalent of Pi-erre, Mr. Ruche's first name. We discover this in the novel when Pierre's old friend from the War (WWII), Elgar, writes to him from Manaus on the Amazon River. First he writes 1001 Pages instead of A Thousand and One Pages for the name of Pierre's bookshop in Paris, then he creates the mathematical equation in place of his name. And, like in The Life of Pi, someone promises the best stories imaginable, only this time the stories are in original editions of math books, or, in the words of Pierre and Elgar, "books of maths". If The Life of Pi contained stories to make you believe in God, then this novel contains stories to make you believe in maths.
[page 2, 3] Dear πR,
From the way I've spelled your name, you should be able to guess who I am. Right first time, it's me, Elgar get your breath back. I know we haven't seen each other for half a century. I've counted. . . .
Why write now, after all this time? Because I've sent you some books. Why you? Because you were my best friend in the whole world, and anyway you're the only bookseller I know. I'm sending you my library: all of my books almost a ton of books about maths.
All the classics are there. I suppose you think it's strange that I refer to maths as if it were literature, but I guarantee that there are better stories in my books than in the best novels. . . . [They] might even make a confirmed reader of novels like you happy. . . .
The books that I have collected were for me alone. Every night, I'd choose one to spend the night with; and they've been wonderful nights, torrid, humid equatorial nights. Nights every bit as important to me as when we were sowing our wild oats long ago in the hotels around the old Sorbonne. . . .Pierre is sure that Elgar is tantalizing him so that he will read the books and want to keep them instead of selling them. "Being and Nothingness" was their college nickname after Pierre wrote an essay on Being and Elgar wrote a thesis on the number zero. Elgar had always insisted that Sartre had stolen their nickname for his famous book. Like Scheherazade with her stories which she told every night for 1001 Nights to stay her execution, Pierre, the man of 1001 Pages, will soon receive almost 1001 books of stories to keep him busy and excited for over 1001 nights. And best of all, he will share what he learns from them each night with the three children of his assistant, Perrette: Max, her youngest, an adopted deaf boy, and his older siblings, the twins, Jonathan and Lea, and of course with us.
The first story of maths is about Thales, the man who gave us the concept of an angle. How fitting it is that we commonly use the Greek letter θ (theta) to represent angles and the first letter of Thales' name in Greek is θ or the digraph "th" in English. What Thales did was to consider that the angle was a thing in itself, thus adding a fourth element to geometry which typically only contained length, breadth, and volume, up until then. Mr. Ruche showed Max, Jonathan and Lea how one might have gone about measuring the height of a great pyramid in Egypt in the time before any maths were available. Mr. Ruche tells the story of how Thales and some fellah he met near the pyramid measured the height of the pyramid.
[page 27] "Thales considered the idea: my shadow relates to me exactly as the shadow of the pyramid relates to the pyramid itself. From this, he deduced that, at the moment his shadow was equal to his height, the shadow of the pyramid would be equal to its height. He had found a solution. Now he simply needed to put it into practice.
"He would not be able to do it alone, so the fellah agreed to help him. At dawn the next day, the fellah went and sat in the shadow of the pyramid. Thales drew a circle in the sand near the pyramid, the radius of which was his height, and stood in the center, keeping his eyes fixed on his shadow. The moment the tip of his shadow touched the circumference, the moment it was equal to his height, he called to the fellah who immediately planted a stake at the point of the pyramid's shadow. Thales ran towards the stake. Using a rope held taut, they measured the distance from the stake to the base of the pyramid. Once they had calculated the length of the shadow, they could begin to calculate the height of the pyramid."This was the first of many stories about pioneers of the maths with which Pierre Ruche regaled his class of three young students, Max (12), Jonathan and Lea (twins of 16), which fills out to four if we include the Amazon blue parrot Max found wounded in the warehouse district. The parrot was named Sid Vicious or simply Sidney. The parrot also seemed to know about maths, but didn't talk very much. The narrator comments after an episode in which Max broadcast, "Attention! Attention! This is a theorem!" through a loudspeaker from a tape recorder:
[page 29] Jonathan and Lea were speechless. Only Sidney seemed unimpressed, perhaps jealous that this non-human was capable of speech. Max pressed "Stop" on the tape recorder and all was silent.
Pierre agreed with what Max had broadcast and called it "Thales' theorem" and explained its importance:
[page 29, 30] "Objects which look similar to each other have the same form. If proportions are conserved, then the form is conserved in fact, you might more accurately say that form is what is conserved when dimensions are changed but proportions maintained."
Thales was apparently learning about a lot more than just angles. Maths have a lot to tell us about life apparently. "Mathematics is simply a series of clever tricks devised by great minds," Pierre said on page 28, and this novel was already in a couple of dozen pages bringing to life one of those clever tricks of the great mind of Thales from 2600 years earlier. Anyone who thinks that maths is what one does with calculators and so one only needs a calculator instead of studying maths is on the wrong track. Try explaining how form is maintained when dimensions are changed using a calculator.
Protagoras is known for his famous dictum that "man is the measure of all things" and that saying takes on new meaning when we discover the unit that Thales used in measuring the pyramid. There were no standard measuring devices such as tape measures or rulers back then, so Thales had to improvise, according to Pierre. His unit shouldn't seem strange to us as our measurement of "foot" is approximately the length of a human foot.
[page 38] "The only thing he had to measure length with was a piece of rope. He needed a unit of measurement, so he used the Thales he measure it in units of his own height."
The large crate of books from Elgar's library undergoes a perilous journey across the Atlantic Ocean to Paris. The tramp steamer was in danger of foundering in high waves and the captain had already given the order to toss the crate of books overboard with the other freight when a Cuban vessel arrived to assist the ship. When it did arrive Pierre had allocated space in an upstairs unused room for it and it came to be known as the Rainforest Library. As he unloaded each book, he found some of them were written before the invention of printing and almost every book was an original or of a very limited edition of a book on mathematics.
Along about this time the men from whom Max wrested the parrot after they fought over who was to get it began looking for Max and the parrot again and they get a hot lead from a pet shop that Max stumbles into. While the lessons in maths are flourishing, sinister forces are endeavoring to extract the parrot from Max's possession. This flux between the stories about maths and the bad guys tracking down Max and Sidney give the novel a pull and keeps the reader engrossed in the parallel plot lines. For me, the stories of the maths provided an excellent review of all the math courses I took from high school all the way through advanced calculus during my academic work in physics. Here were stories about such men as Fermat, Gauss, Dekekind, Decartes, Cantor, Napier, Legendre, Lagrange, Pascal, Riemann, Euler, and many other familiar names which I only knew from studying how to use their "clever tricks," but about whose lives I knew very little. To me this was the fascinating attraction of this book which kept me turning page after page in delightful anticipation. For example, I was amazed to find Euler wrote over 600 books on mathematics. No wonder his name is attached to so many aspects of maths. The Euler Equation utterly amazed me and delighted me when I first saw it. The five most important constants in the history of the word all combined into one simple equation. I committed to memory that very first day back in the 1960s and can recite it or write it without hesitation, but I had forgotten the most important detail, the name of the man who originated it, up until now. This is how his equation reads: (e to the power of (pi times the square of -1)) plus one equals zero. Symbolically one can see the five great constants of all mathematics interlocked in one great embrace, thanks to Euler:
Euler's Equation: eπi + 1 = 0
When it came to Arabic mathematicians, I knew very little except that our word "Algebra" came from some Arab's name. I was surprised to find that the famous mystic and poet Omar Khayyam was a great algebra man.
In what is a finely researched and executed history of mathematics, I could only find fault with one claim, which happens when Pierre says that the Pythagoreans thought the Music of the Spheres was a musical scale. This is a gross, materialistic misrepresentation of what the Pythagoreans understood as the Music of the Spheres. To the ancient people in Pythagoras's time, they could directly perceive the Music of the Spheres as a spiritual reality. Here is what Pierre says:
[page 82] Mr Ruche continued. "Pythagoreans believed that the whole universe was series of harmonies, that the heavens themselves were regulated by a musical scale. They called it the Music of the Spheres.
Ruche is describing the beliefs that materialistic scientists have retrofit upon the Pythagoreans and ancient Greeks which still possessed a spiritual ear. Read now about what those people actually experienced as a spiritual reality, a reality which is no longer commonly experienced today due to an evolution of consciousness which has brought about a devolution of the direct experience of spiritual realities, a loss of our spiritual ear.
[page 93 of Theosophy by Rudolf Steiner] Besides what is to be perceived by spiritual sight in this Spiritland, there is something else that is to be regarded as spiritual hearing. As soon as the clairvoyant rises out of the soul-world into the spirit-world, the archetypes that are perceived sound as well. This sounding is a purely spiritual process. It must be conceived of without any accompanying thought of physical sound. The observer feels as if he were in an ocean of tones. And in these tones, in this spiritual sounding, the beings of the spirit-world express themselves. The primordial laws of their existence are expressed in their mutual relationships and affinities, in the intermingling of their sounds, their harmonies, melodies and rhythms. What the intellect perceives in the physical world as law, as idea, reveals itself to the spiritual ear as a spiritual music. (Hence, the Pythagoreans called this perception of the spiritual world the Music of the Spheres. To one who possesses the spiritual ear this Music of the Spheres is not something merely figurative and allegorical, but a spiritual reality well known to him.)
By no means did the Pythagoreans think of the heavens as regulated by a musical scale called the Music of the Spheres. The heavens they referred to were the spiritual realities which they perceived with their spiritual ear and that reality they called the Music of the Spheres. The other meanings of this phrase have been generated over the centuries as our ability to perceive this reality gradually died away until few people today are able to perceive it.
Rudolf Steiner was able to perceive this reality and his writings enable us to understand correctly what the Greeks meant by the Music of the Spheres. This understanding is today available as an esoteric knowledge through Steiner's spiritual science or anthroposophy. A few pages later, Pierre Ruche explains what it meant to have exoteric and esoteric knowledge to Pythagoras' students. He explained that Pythagoras divided his schoolroom with a curtain.
[page 84, 85] "Pythagoras would sit on one side and the candidates on the other. They could listen, but could not see. This period lasted for five years. . . . The curtain played an important role in the Pythagorean school. To go beyond it meant that one had passed the tests. Those on the far side of the curtain were called exoterics and those who sat with Pythagoras were esoterics. They alone could hear and see him."
Those who can directly perceive the spiritual world today are called esoterics, and the rest of us sit on the outside of the curtain as exoterics. We can only discuss what the esoterics experience directly. But if we listen carefully to what the esoteric Rudolf Steiner says, even though we have a curtain between us and the spiritual world, a world that he can perceive directly, we can come to understand the spiritual realities of that world, a world which would otherwise remain a mystery to us. If we have no access to esoteric knowledge, all mystery could be removed from us by exoteric teachers who would replace it with flattened, dry, and abstract exoteric knowledge of the materialistic portion of the world which they claim is the only world there is.
The reality of the Music of the Spheres is no small thing. It is certainly not merely an abstract musical scale.
Albert the taxi driver was a guide to the world, a world which he learned about from his passengers who were world travelers. He knew of the cities of the world, New York, Tokyo, Bogota, Singapore, Rio, all without leaving his cab. To Albert cities were a reality and countries an abstract concept, a figure which only exists on a map.
[page 91] "Cities, mind you, not countries. Countries only exist on maps, but cities . . . cities are real places."
Numbers were real things to the Greeks. By numbers they meant integers. They had no word to distinguish numbers from integers because all the numbers they knew of were integers. The very word "integer" is a modern concept which had to be introduced after the Greeks worked through the play of concepts which might be called the "Square Root of 2 in 3 Acts". It is a drama which shaped the very world we live in today.
[page 92] "Since some of you can't wait to find out about how irrational numbers rocked the world more than two thousand years ago, we've organized this night school", began Mr Ruche. "It was in the fifth century BC, in a town called Crotona somewhere in the Greek Empire probably what we now call southern Italy, and it's a drama in three acts. Act 1: Everything is a number. Act 2: If a square has sides one unit in length, the diagonal of the square cannot be expressed as a number. Act 3: Therefore measurement must exist which cannot be expressed as numbers. This idea, which the Pythagoreans put forward themselves, suddenly placed their view of the world in jeopardy. It was essential that it be kept secret."
In this dramatic episode we see how esoteric knowledge began. Those who had the knowledge felt that the rest of the world was not ready to receive it and that it had best be kept secret until the world were ready. Rudolf Steiner made it clear that the esoteric knowledge he possessed, which had been kept secret from the public for ages going back before ancient Greece, was knowledge that the world was ready to receive at the beginning of the twentieth century, and he presented his esoteric knowledge in his writings and lectures as a spiritual science. He called it a science of the full human being or anthropos and gave it the name, anthroposophy.
Everyone has heard of the famous library of Alexandria, but how many knew of the impact of that city upon the world for seven centuries?
[page 100] Mr. Ruche went on: "Cities everywhere were clamoring for status, but it was Alexandria that took the place of Athens. For seven hundred years Alexandria was the center of intellectual thought of the Western world."
Another bit of information about the origin of the word "volume" used to apply to books. One of the Rainforest Library books came in scroll form. Mr Ruche is explaining to his students how the library of Alexandria often kept originals and had scribes create a copy to return to the owner. Jonathan exclaims, "That's a swindle!" and Ruche replies. But as we can tell, the copies were on new papyrus and probably came out looking better than the originals.
[page 103] "Well, that's the way it was", said Mr Ruche. "They were hardly cheap copies though. Both the originals and the copies were written out on rolls of papyrus. The first manuscripts were kept in rolls in Latin, volumen, hence the word 'volume'."
On page 110, Mr Ruche explains how to find the lowest common multiple and the highest common factor of two numbers. His example was for 12 and 15 (60 and 3), and I tried my hand at it for 18 and 21 and got 126 and 3. On page 112 and 113 he describes how the four basic elements of the ancient peoples were related to the complete set of regular polyhedrons: fire tetrahedron, earth cube, air octahedron, and water icosahedron. The fifth regular polyhedron, the dodecahedron, Plato related to the cosmos because it was the nearest to being spherical in shape.
Add to the number of catastrophes created by a love affair gone bad the loss of the Library of Alexandria. When Cleopatra's marriage to her brother, Ptolemy XIII, went bad, she fled Alexandria for Rome with Julius Caesar and they set fire to the ships in the port to expedite their escape. That fire spread to the Library and thousands of originals and irreplaceable volumes were burnt and disappeared from the world forever. (Page 121)
Meanwhile the Roman Empire which filled the world with its oppressive law and modeled its art and literature from the Greek civilization, left only its juvenile addition to mathematics, its Roman numbers which are almost useless for any mathematical work. They appear primarily on clock faces, in outlines, and to mark chapters of books today. Mr Ruche comments wryly, "In a thousand years of the Roman Empire, there is not a whisper of mathematical thought." (Page 123)
If anyone needed proof that the Greeks were master mathematicians, how about this: they invented the concept of a proof!
[page 127] "Unlike the Egyptians and the Babylonians, the Greeks refused to accept that intuition was enough to support mathematical truths. They also refused to accept specific examples as proof: I believe because I see, you believe because I show you. This was the "proof" accepted on the banks of the Nile and the Euphrates. Greek mathematicians were not satisfied with this: they wanted an argument, a proof."
"Didn't that exist before them?" Lea asked, surprised.
"No, the Greeks invented it." Mr Ruche added the rice to the shallots and stirred until the grains became translucent. He kept stirring, careful not to let the grains stick together. When he had a rhythm going, he went on: "But turning your back on intuition and on empirical evidence means there is always a doubt. If seeing is not believing, then how can I ever state that something is true? How can I convince you, or even myself, that what I say is true? This raises the most important questions the Greeks asked themselves: How do we think? Why do we think what we think? How do I prove that what I think is true?"Of course there is a limitation in the method they used for proof. We find a story or play in two acts. Act 1 states the principle of non contradiction: "A statement and its opposite cannot both be affirmed." Act 2 states the principle of no third: "An assertion and its opposite cannot both be false." "If one is false, the other must be true, there is no other possibility." (Page 128) These are the basic premises of Aristotelian logic which served science well for over 2,000 years. The limitation is that life itself is seldom so simple as to require one or another proposition to be true and the other false. Those propositions are maps of reality and Alfred Korzybski spent his life showing us that the map is not the territory and that anyone who mistakes the map for the territory acts from an un-sane position. His landmark book which provided the basis for the science of General Semantics is titled Science and Sanity and is still in print today, 74 years after its publication in 1933. Its subtitle explains clearly that his approach to science and sanity operates outside strict Aristotelian principles: "An Introduction to Non-Aristotelian Systems and General Semantics".
One might get a chuckle as I did from the idea that geometry originated as writing did: as a way of calculating the amount of one's physical property, usually for tax purposes. In this next passage, it is the girl twin, Lea, who explains how geometry began as a way of calculating taxes in Egypt. As a famous French saying translates, "The more things change, the more they stay the same."
[page 131] "I'm sure you know," Lea continued, "that according to the Greek historian Herodotus, the true beginnings of geometry were in Egypt. In 2000 BC, Ramses II decided to give each of his subjects a plot of land of equal size so that they would each have to pay the same taxes. However, every year the Nile burst its banks and some plots would lose ground, so that they could calculate the percentage of the tax to rebate. This was the true beginning of geometry."
If you've ever wondered what the big deal was about "squaring the circle", duplicating a cube, or trisecting an angle, this book will enlighten you as to why the difficulty and how some of the solutions violated the principles required for a proper solution.
If you have never heard of the Institut du Monde Arab, it was constructed from 1981 to 1987 in Paris and is covered with computer-controlled irises to adjust automatically the amount of light coming into the building. Below is a closeup of one of the active sun control diaphragms. Below is a passage where Albert the taxi driver drops off Mr Ruche at the IMA.
[page 145] Mr Ruche closed the Rubaiyat and opened a biography of Omar al-Khayyam. He was deeply engrossed in his reading when he heard a whirring sound. He looked around, but couldn't see anything. The sound continued and his eye was caught by the great glass façade. What he saw astonished him. A metal shutter, like the iris of an eye, surrounded each window and they were all closing slowly. It lasted no more than a minute, then the noise stopped the shutters were almost closed.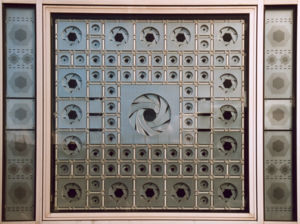
The young woman who had helped Mr Ruche could not help but laugh at his astonishment. 'There are 27,000 of them exactly,' she said, explaining that there were 240 panes in the façade, each with more than a hundred shutters. A photoelectric cell linked to a computer regulated the light that flooded into the building. When the sun was too bright, the shutters closed, like a squinting eye. She was studying architecture and had come to see how the building worked. The young woman pointed out that each pane represented the classic elements of Arabic geometry. She showed the delighted Mr Ruche that the figures moved round in rotation, the architect having cleverly combined squares, circles, octagons and stars.Another amazing revelation is that what we commonly call "arabic numbers" originated in India and should more accurately be called "Indian symbols" to credit the originating country. In 733 a book written a century earlier in India by Bramagupta called Brhmasphutasiddhntha arrived in Baghdad and was immediately translated into arabic as the famous book Z j al Sind-hind. Mr Ruche is talking.
[page 154] "It revolutionized science in the Arab world through ten little symbols that every one of you knows well. The ten numbers we use to calculate: 1, 2, 3, ... up to 9, not forgetting the last "zero".
"The man who brought the book knew the symbols and had used them for years to calculate. He had changed them so often on the journey to the Round City that everyone in the caravan knew them by heart. At night, around the campfire, one of them would begin and the others would start to sing out the numbers to the dark."
In the darkness of the library, Sidney's voice rang out: "Eka, dva, tri, catur, pance, sat, sapta, asta, nava."
What about zero? asked Lea.
"Sanya", said Mr Ruche, who had kept the honor of introducing "zero" for himself.
There was a long drum roll.
"Sanya means 'empty' in Sanskrit. Zero was represented by a little circle. "Empty" translated into Arabic becomes sifr which in Latin becomes zephirum, which in turn becomes zephiro in Italian, and from there it's a short step to zero."I daresay you can not read a more complete etymology of zero anywhere else. Every other number represented some thing that was full, like a basket of apples could have 1, 2, 3, etc apples in it, but this magical number zero, one of the five great constants in Euler's Equation above, could represent an empty basket for the first time in the history of the human race! As simple as it was profound, this creation of the number for an empty basket followed a process that would lead to the creation of the other three constants in Euler's Equation, namely, π, e, and i. The process was to notice that if a constant came up in certain form of equations, such as the square root of minus 1, it would be advantageous to pretend it exists and create a symbol, such as i, to represent it. The four constants which fill out Euler's Equation all came into being in a similar fashion: 0, π, e, and i.
The Indian number system added something else: positional notation. This was hitherto unknown. In Roman numerals, an X represents the number 10 no matter where it appears. XXX = 30, but the number 30 in the Indian number system is represented by moving the 3 to the left one place and putting the zero symbol to hold the place or position of the number. Thus, a 3 in the left position represents three times 10 or thirty items and in the right position represents only three items.
Everyone in the world uses this form of positional notation in whatever number system they use. In base 2 or binary number systems we use only 0 and 1, in octal we use 0, 1, to 7, and in hexadecimal we must add six new symbols to fill out the sixteen characters. Since hexadecimal (base=16) number system is used for computer work, it was essential that we utilize already existing symbols which had an order to them, so we used the first six letters of the alphabet to represent the numbers 10 through 15: a, b, c, d, e, f. The number sixteen is naturally shown by a 1 in the sixteens position and a 0 in the ones position, or 10. The rules are the same, no matter what base number system you operate within. Number systems such as base 2, base 4, base 8, base 16 are easy for binary computers to calculate with, so all data is converted into binary for calculation and back into our normal base ten for printing and other human, non-computer uses. I recall a three year project I worked on where I spent hours each day over machine code printouts deciphering data and addresses in the octal number system. At the same time I noticed a high rate of errors in balancing my checkbook because I would inadvertently, e.g. add 7 + 3 and come up with 12, which is correct for octal addition, but not decimal. When I switched to hexadecimal in my next computer job, this error-prone quirk disappeared.
Someone who fixes bones is known as an "algebrist" from the arabic word, "al-Jabr" which means "bone-setter." That is the origin also of our word "algebra" for the arithmetic process by which we fix what is broken by solving for the unknowns in equations. When we have a solution to an algebraic equation we can "set the bones" all in front of us for everyone to see, i.e., lay out the values of the unknowns which satisfy the requirements of the equations before us. Like a doctor who wishes to set a broken bone must probe the unknown condition of the bones inside of someone's limb before setting it straight, so also must an algebraic physician probe the unknown to set the matter straight for his equations. Mr Ruche explains to his rapt students.
[page 157, 58] "In algebra a little like bone-setting you move things from left to right, trying to find what is missing, said Mr Ruche. This is how al-Khwarizmi explains it: 'That which I am searching for, I begin by naming. But since I do not know what it is; since it is this that I seek, I simply call it the thing.' This is the unknown what mathematicians might now call x or y. By naming the thing, as he calls it though he does not have a value for it he can work with it as he does with other numbers. His strategy is to try to calculate an unknown value by treating it as though it were known. He adds to it, subtracts from it, multiplies and divides, but in doing so he is trying to find out what it is. Discovering the unknown is the magic of algebra."
Now this ancient Arab had still a lot of problems when he encountered negative roots, fractions, and irrational numbers and did not know how to deal with them. So he dealt with them as a bone-setter might. He called negative numbers "amputated", fractions "broken", and irrational numbers "deaf". (Page 158)
Ah, books! In this next passage, the Denis Geudj has Mr Ruche describe books in a way that only bibliophiles can really appreciate.
[page 165] "Books do not bring the dead to life", read Mr Ruche. "They cannot make a madman sane, nor a foolish man wise. Books sharpen the mind; bring it to life, quench its thirst for knowledge. But for he who would know everything, it would be better that his family take him to a doctor, for this can come only from a troubled mind.
"Dumb when you wish it to be silent, eloquent when you wish it to speak, a book can teach you more in a month than the wisest men can in a lifetime and without a debt owed for knowledge gained(2). Books make a man free, deliver him from dealings with the odious, the stupid and those who cannot understand. A book is obedient, whether a man travel, or whether he be still. If you are disgraced, a book will serve you no less well. If others turn against you, a book will never turn its back on you. Sometimes a book may even be greater than he who wrote it."In the 1950s a new toy named Etch-a-Sketch(tm) came out which allowed kids and adults to write or draw pictures on a board covered with dust by running stylus across the back of the board. Everyone thought, "What great new technology this is! All you have to do is shake it to erase the board and start over." I wonder what they would have thought if they'd known that early Indian and Arab mathematicians were carrying such a board around with them and simply sprinkling sand or flour on the board to write out their calculations. "Numbers written in this way were often known as 'dust numbers'." (From footnote on page 166)
Another surprise was that the appearance of the minus sign preceded that of the plus sign. Also a surprise that both these signs did not come into use until 1489 when Johan Widman designed them to help him manage his crates of merchandise, noting when one was over weight or underweight. Once more the businessman becomes an innovator in number systems and mathematicians adopt his innovation by a matter of circadian necessity. Jonathan is talking here, explaining how the + and - signs originated in a business practice.
[page 196] "It was in 1489 and the author, Johan Widman, used them to mark his crates of merchandise. The crates were called largels and once filled, each supposed to weigh 4 centner. When the crates did not come to the exact weight if one was five pounds light, for example he would mark it "4c - 5l". If, on the other hand, the crate was five pounds heavier, he put a cross through the symbol, making "4c + 5l". From the wooden crates, the symbols made their way into his accounting books, and from the world of business they found their way into the world of algebra."
Everyone should know that the Greeks used masks to hide their faces when performing on stage. They spoke through the masks and their words for speaking through per-sona gave us our English word, persona. But what I was not aware of, until this book, was that the actors used the masks to also hide their own reactions to the other actors and when speaking their own lines. Today actors do not hide their emotional reactions to the lines, but rather they use their natural reactions to amplify the effects of the lines! This next passage is from Descartes's opening lines in "Rules for the Guidance of the Mind."
[page 227] Actors upon a stage wear masks to hide their blushes. As I set foot upon the world stage for the first time, having hitherto been a mere spectator, like them I advance masked.
As an inveterate margin scribbler, I can appreciate the difficulty Fermat had in writing down the proof of his theorem that it is "impossible to separate a cube into two cubes" or "any higher power except a square into two powers with the same exponent." (Page 246) Here's what Fermat added in the narrow margin of Bachet's translation of Diophantus, Book II, opposite Problem 8.
[page 247] I have discovered a truly marvelous proof of this, which, however, the margin is not large enough to contain.
How many times it has happened to me that the margins of the book I was reading required me to make do with a brief summary of what I wished to write out in toto and later to find myself spending much time trying to decipher my tiny script which was made impossible to read due to the width of the pen stroke combined with the lack of space in the margin. Often I resort to using the blank space at the end of chapters or the blank end pages to flesh out my longer discourses, notes, or poems. I loved the library as a child and college student, but as an adult I can not be restricted to read-only books, but require that the books I read be capable of my writing in them. Like Fermat, my books contain scraps of my ideas, thoughts, notes, poem ideas, or complete poems which will possibly be to future generations of value. For reasons of establishing priority in some idea or other primary property, I also affix the date at which I read and write in the margins of any book in a unique way that would make it unlikely for someone to back date some notation. This involves a special date glyph which I design for each new year when it arrives. By the time I am writing these glyphes in my books, I have developed a smooth stroke for their execution (especially after the first month) that would be difficult to imitate successfully. Each glyph is thus like a date-based signature. I can sign and date a document with two continuous strokes.
In the study of π we find that there is π in the sky and π on the Earth. Most of my life I have lived within a few miles of the large and winding Mississippi River which is governed by the curious number we know as π. During their field trip to the Palace of Discovery built for the Great Exhibition of 1900, a large oval room was built with the first 700 decimals of π emblazoned in a complete arc around the room. They listened to the guide talk about π and how it could be found in the far reaches of the cosmos as well as down here on Earth.
[page 263] "Two or three little things before we finish', said the guide. 'You shouldn't think that π exists only in the world of pure maths. It can also be found, here and there, in physical phenomena and in cosmology." He pointed to the dome above and pressed a button. The lights went out, and a projection of the stars appeared.
"Some astronomers believe that π is present in the heavens. If every star in the sky is referenced by two coordinates expressed as whole numbers, the probability that those numbers will have no common factor is 6/(π2).
The lights in the dome came on again.
"Here on earth, π is linked to the great rivers of the world", the guide continued. "Large rivers flow slowly and tend to meander. If one compares the length of the river from source to estuary as the crow flies with the true length of the river, measuring all the meandering, the ratio is almost exactly 3.14. The flatter the topography, the closer the ratio is to π.We know a lot about π, but what about this base of the natural or Naperian logarithms, e what do we know about it? It seems that if you invest money compounded yearly at 100 percent interest, you'll get exactly 2 times your initial investment after a year. Here we go into business mathematics again to discover one of the most useful constants for scientific work. If you can arrange to get your interest compounded twice a year, you'll get more money, right? So far, so good. But suppose you arrange to have it compounded every nanosecond, that is a billion times a second how much would your investment be inflated by the end of the year? 3 times, 10 times, 100 times? Well, the answer is simple: you would receive e times (2.716..., or under 3 times) your initial investment! (Page 265) In mathematical physics one is relieved to find that the derivative of ex is ex. What a relief compared to the other derivatives whose formulae one has to memorize. What does this mean that the derivative of ex is itself? It means that on the curve of ex every point is rising at a rate equal to e raised to power of the value of that point on the curve. Takes a while to wrap your mind around that one, but it shows how unique the constant e is.
As far back as 1775 the Royal Academy for the Sciences in Pari had decided that it would no longer accept for consideration solutions to the problems of squaring the circle, trisecting an angle or duplicating a cube, and "any machine said to have perpetual motion." (Page 280) I had a personal connection to the fourth problem when I assisted Joseph Newman in describing his energy machine which had all the attributes of a perpetual motion machine because it created more energy than it required to power it. We found that his theory involved extracting of electricity directly out of the magnetic field wires and we hypothesized that this additional energy came from direct conversion of copper into energy by some hitherto unknown process. But Newman has had an uphill battle acquiring a patent for a machine which seemed to fit the specifications of the type of machine disdained by the Royal Academy for over 200 years. Some 25 years later, Newman is still building larger and larger energy machines which offer the promise of limitless energy by direct conversion of mass into energy without splitting or fusing atomic particles. The spirit of the Royal Academy apparently has long arms extending over the world and over time.
Max and his parrot, Sidney, are kidnaped and Pierre Ruche must drive in Albert's taxi from Paris to Sicily to find them and when they arrive they find that Pierre's other old friend, a third pal from the war, is waiting to greet them. So the mystery is solved, but with great difficulty and we can only wonder if the parrot will live happily ever after or die at the hands of the kidnappers.
What have we learned from our journey through the history of maths? Deep gratitude to the men whose lives were devoted to coming up with the "series of clever tricks" we know as mathematics. A deeper respect for the contributions of the Arabs, for another thing. They took a book written in India with the ten digits we all use now, translated it, and made it into such a popular form of calculating that it was their name that popular parlance has attached to what we call "Arabic numbers." Then Al-Jabr began a little bone-setting and we had algebra to help us set things straight in a world of unknowns. We have also learned that maths can be exciting. Denis Guedj orchestrates the lives of Elgar, Mr Ruche, Perrette, Albert the taxi driver, Max, the teenage twins: Jonathan and Lea, an Amazon blue parrot name Sid Vicious, and a few thugs to convey the sense of excitement that anyone can get by studying the history of mathematics. We have a chance to visit the Institu du Monde Arab building and watch the 27,000 irises open automatically to let more light into the glass monolith and walk through the Palace of Discovery with the large room with the 700 digits of Pi arrayed around its lower ceiling. We have learned the origin of the five great constants of π, e, i, 0, and 1 and why they are important even to people who have never heard of some of them, and how they are united in one grand embrace in Euler's Equation. If five such amazingly different numbers which came into existence over thousands of years can be related so intimately it gives us hope that the amazingly different people of the world will discover that we are all intimately related to each other and can embrace each other as well.
~^~
---------------------------- Footnotes -----------------------------------------
Footnote 1. See The Life of Pi by Yann Martel, the story of a boy on a lifeboat with a 450-pound Bengal tiger.
Return to text directly before Footnote 1.
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~Footnote 2. In my opinion, we are never without a debt owed for knowledge, however gained. When we gain knowledge from someone who originated it or thought to pass it on, we have acquired their primary property, and we have a moral duty to use it always with a sense of obligation to the person from whose life these thoughts and ideas have originated. My understanding of this basic principle of right thinking I owe to Andrew Joseph Galambos and his teachings in the Volitional Science course, V50, which are now embodied in Sic Itur Ad Astra.
Return to text directly before Footnote 2.
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
~^~
Any questions about this review, Contact: Bobby Matherne
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Click Left Photo for List of All ARJ2 Reviews Click Right Bookcover for Next Review in List Did you Enjoy this Webpage?
Subscribe to the Good Mountain Press Digest: Click Here!
CLICK ON FLAGS TO OPEN OUR FIRST-AID KIT.
Do you feel like you're swimming against a strong current in your life? Are you fearful? Are you seeing red? Very angry? Anxious? Feel down or upset by everyday occurrences? Plagued by chronic discomforts like migraine headaches? Have seasickness on cruises? Have butterflies when you get up to speak? Learn to use this simple 21st Century memory technique. Remove these unwanted physical body states, and even more, without surgery, drugs, or psychotherapy, and best of all: without charge to you.
Simply CLICK AND OPEN the
FIRST-AID KIT.
